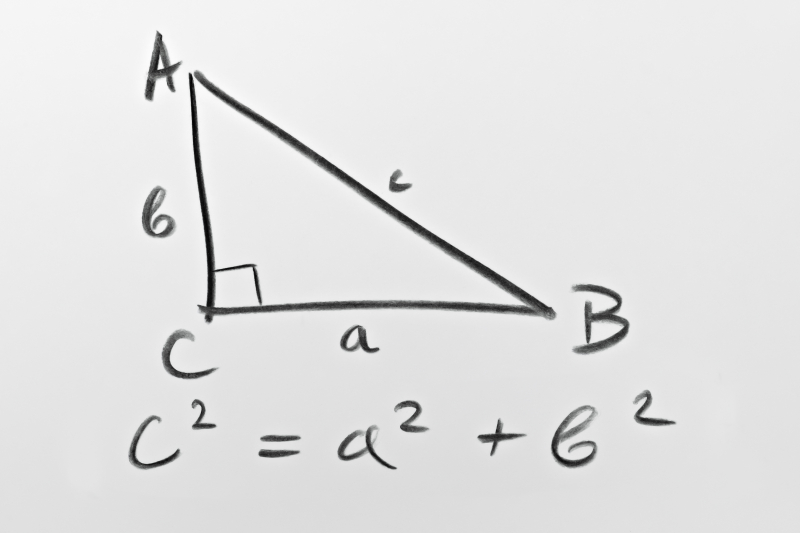Basis of geometry

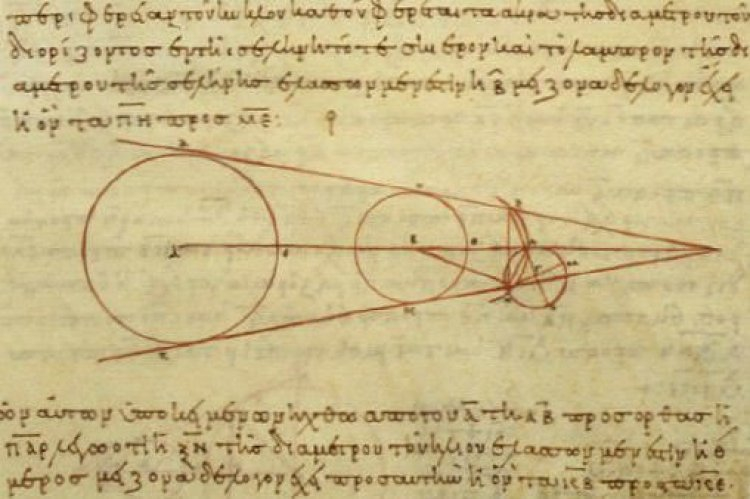
One of the oldest branches of mathematics, probably even older than arithmetic itself, is geometry. And even before any of these geometric methods were known to history, its practical need compelled their employment. Many of these techniques were developed and used for the first time by the Egyptians, Babylonians, and Indus people, but they were never able to discover the laws and axioms of geometry. The Babylonians accepted the value of Pi as 3, never questioning its correctness. The Ancient Greeks were avid students of geometry despite not having invented it. The Greeks used what had been learned and made significant improvements that helped to define modern geometry. In fact, learning geometry was regarded as the pinnacle of mathematics and science in Ancient Greece.

When Greek geometry emerged, everything changed. Similar to how it is done now, the Greeks emphasized that geometric facts must be proven by deductive reasoning. The inventor of geometry, Thales of Miletus, provided several axioms and laws that were actually founded on logic and were known as mathematical truths in the sixth century BC. Then came the likes of Pythagoras, Euclid, and Archimedes, the axioms and laws of whose geometry are still taught in classrooms today. Even though there were many more Greek mathematicians and geometrists who made contributions to the history of geometry, these figures are the actual giants since they are responsible for creating geometry as we know it today.